What’s the Force of a Baseball Bat Swing by Mike Trout?

by

The sheer force of a baseball bat swing striking a ball by Mike Trout, one of the MLB’s elite players, is tremendous.

Join me on this exhilarating adventure as we crunch some numbers and calculate the Herculean force he generates when smashing a line drive in the MLB.

Calculation variables for determining average force on a bat during a collision

First and foremost, we’ll be concentrating on the crème de la crème of MLB players. So, let’s dive into some batting stats from MLB superstars.

According to Insider Baseball, while the average exit velocity for a baseball in the MLB hovers around 89 mph, Trout has been recorded smashing a line drive with an exit velocity of a whopping 118 mph!

The MLB also provides another interesting stat: the average speed of a fastball pitch is about 92.3 mph. For our calculations, we’ll be considering a few more essential variables:

  • A baseball weighs: 5.125 ounces (0.145 kg)
  • The contact time between the baseball and the bat is a mere 0.7 milliseconds (0.0007 seconds). Crazy, right? The magic happens in a blink of an eye.

But before we jump into the calculations, let’s put a little disclaimer here: our calculations will rely on some assumptions due to the unavailability of complete data about Trout’s remarkable hit and the details of the pitcher.

Calculating the average force on the baseball bat during the collision

mike trout hitting baseball in game
Mike Trout swinging (Photo Credit: Erik Drost)

Our mission begins with the calculation of the change in momentum of the ball. Once we have that, we can determine the average force exerted on both the bat and the ball during the ultra-short collision.

Important Note: During a collision, momentum gets transferred. The momentum of a ball depends on its mass and the velocity it carries. When a batter swings, they convert their potential energy into kinetic energy, which gives the bat momentum. Upon contact, the bat and ball exchange momentum, all while adhering to the Law of Conservation of Momentum—meaning the total momentum remains constant.

The change in the ball’s momentum

Picture a baseball zinging through the air. Momentum plays a massive role in this game of speed, force, and physics. Momentum, which we define using the equation p = mv (where ‘m’ is mass and ‘v’ is velocity), is at the heart of this baseball dance. Let’s dig into how the momentum of a baseball changes when it’s on the move.

A baseball traveling in a straight line is subject to a force, let’s call it F = F(t), that varies over time. Over a particular time span – say from (t_{0}, t_{1}) – the momentum’s change equals the integral of F from t_{0} to t_{1}. In other words:

p(t_{1}) - p(t_{0}) = \int_{t_{0}}^{t_{1}} F(t)dt

So, this integral shows the force’s impulse during that period. Now, if we’re calculating the momentum shift, we use the formula F = ma = m\dfrac{dv}{dt}, where ‘a’ signifies acceleration and ‘v’ for velocity. That leaves us with this equation:

\int_{t_{0}}^{t_{1}} F(t)dt = \int_{t_{0}}^{t_{1}} m(\dfrac{dv}{dt})dt = m\int_{v_{0}}^{v_{1}}dv

= (mv)|_{v_{0}}^{v_{1}} = mv_{1} - mv_{0} = p(t_{1}) - p(t_{0})

Now, for the fun part, we’re going to figure out the baseball’s momentum change. Here’s how it looks:

First, we convert the velocity of the ball from miles per hour to feet per second for v_{1} and v_{0}:

v_{1} = 118 \: mph = \dfrac{118 \: mile}{1 \: hour} \times \dfrac{5280 \: feet}{1 \: mile} \times \dfrac{1 \: hour}{3600 \: seconds} = 173.1 \: feet/second

v_{0} = -92.3 \: mph = \dfrac{-92.3 \: mile}{1 \: hour} \times \dfrac{5280 \: feet}{1 \: mile} \times \dfrac{1 \: hour}{3600 \: seconds} = -135.4 \: feet/second

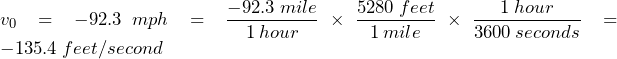
Notice the plus and minus signs on these velocities. The plus means the ball is flying away from the batter after the hit, while the minus signifies it’s hurtling towards the batter after the pitch.

We then convert the baseball’s mass to slugs. And in case you’re wondering, 1 oz = 0.00194 slugs:

\dfrac{5.125 \: ounces}{1} \times \dfrac{0.00194}{1 \: ounce} = 0.0099 slugs

With our mass and velocities figured out, we finally calculate the change in momentum:

mv_{1} - mv_{0} = m(v_{1} - v_{0}) = p(t_{1}) - p(t_{0}) = 0.0099 \times [173.1 - (-135.4)] = 3.05 slug-feet

Calculating the average force on the bat

Time to shift gears and dig into the force that the bat encountered during Mike Trout’s legendary line drive hit. We’ll use the integral from earlier and the calculated change in the ball’s momentum. Crunching the numbers, we get:

\int_{t_{0}}^{t_{1}} F(t)dt = \int_{0}^{0.0007} F(t)dt = p(0.0007) - p(0) = 3.05

So, over a microsecond interval from 0 to 0.0007 seconds, the average force amounts to:

\dfrac{1}{0.0007} \int_{0}^{0.0007} F(t)dt = \dfrac{1}{0.0007} \times (3.05) = 4,357.1 pounds of force.

What happens if Mike Trout hits the fastest recorded pitch speed?

mike trout hitting ball
Mike Trout hitting ball (Photo Credit: Erik Drost [image cropped])
Back in September 2010, Aroldis Chapman catapulted himself into the history books by hurling the fastest pitch ever recorded in MLB – a fiery 105.1 mph.

Now, imagine the raw power that would be unleashed on the bat as Trout swings at this ballistic pitch!

Dr. Alan Nathan has stated that every 1 mph increase in pitch speed causes the exit velocity to jump by 0.2 mph. Sure, it might sound too simple, but hey, it’s the best info we got!

Just remember, as the pitch speed cranks up, making solid contact with the ball gets trickier.

Despite this, let’s assume that Trout nails the contact just like in our initial calculation, even with the speed demons being hurled his way. And we’ll also assume that the contact time remains the same at 0.0007 milliseconds.

Considering all the assumptions we’ve made, Dr. Alan Nathan’s exit velocity formula seems like our best bet. Our pitch speed has now rocketed from 92.3 mph to 105.1 mph, an increase of 12.8 mph.

According to Dr. Nathan, this would crank up the exit velocity by 2.56 mph. Adding this to our initial 118 mph, we hit a thrilling exit velocity of 120.56 mph. Round that up, and we have an adrenaline-pumping exit velocity of 120.6 mph!

Calculating the average force on the bat

Now, it’s time to use this new value and calculate the average force on the bat. Here we go:

v_{1} = 120.56 \: mph = \dfrac{120.56 \: mile}{1 \: hour} \times \dfrac{5280 \: feet}{1 \: mile} \times \dfrac{1 \: hour}{3600 \: seconds} = 176.8 \: feet/second

v_{0} = -105.1 \: mph = \dfrac{-105.1 \: mile}{1 \: hour} \times \dfrac{5280 \: feet}{1 \: mile} \times \dfrac{1 \: hour}{3600 \: seconds} = -154.1 \: feet/second

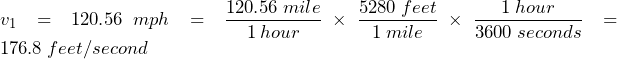
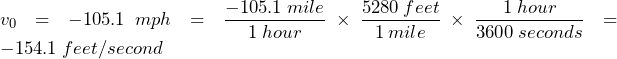
Now we’ve got to consider the mass of our baseball in good old slugs again, knowing that 1 oz = 0.00194 slugs.

\dfrac{5.125 \: ounces}{1} \times \dfrac{0.00194}{1 \: ounce} = 0.0099 slugs

mv_{1} - mv_{0} = m(v_{1} - v_{0}) = p(t_{1}) - p(t_{0}) = 0.0099 \times [176.8 - (-154.1)] = 3.28 slug-feet

Taking that value and integrating it over time:

\dfrac{1}{0.0007} \int_{0}^{0.0007} F(t)dt = \dfrac{1}{0.0007} \times (3.28) = 4,685.7 pound-force.

That’s a lot of force! And guess what? That increase in pitch speed adds a whopping 328.6 pound-force.

But let’s not stop there. If we convert 4,685.7 pound-force to Newtons, we get a jaw-dropping 20,843 Newtons.

For a fun comparison, I crunched the numbers for the force behind Zion Williamson’s epic vertical jump, and it came to a mere 5,134.25 Newtons.

So, it’s clear as day: the collision between bat and ball is a force to be reckoned with!

Conclusion

I’m sure we can all agree that there’s something electric about Mike Trout. He’s not just a major player in the MLB. No, he’s so much more than that. If you ask me, he’s one of the most extraordinary athletes of our time.

Picture this: the raw power he pours into every swing, the bat connecting with the ball in a magnificent display of strength. It’s simply astonishing. Now consider this widely accepted notion – that hitting a baseball is probably the toughest skill to nail down in all of sports. Put the two together, and it’s enough to make your jaw hit the floor.

With Trout, we’re witnessing a blend of phenomenal hitting technique and primal, raw force. It’s akin to a flawlessly executed symphony, each note ringing true and clear. This is where our number-crunching session reveals a critical detail: how the speed of a pitch plays a huge role in the force generated by a hit. Oh, and let’s not gloss over another vital factor—the speed of the bat swing. Although we didn’t factor it into our calculations, it’s a key player in the grand spectacle of baseball.

In the end, baseball is teeming with mind-blowing physics, which is one of the many reasons why I reckon it’s such a kick-ass sport!

What do you think about the force of a baseball bat swing? Got any predictions about its future? Will we see an increase in the force of a baseball bat swing as time moves forward?

Featured Image Photo Credit: Erik Drost (image cropped)

SUBSCRIBE TO ENGINEER CALCS NEWSLETTER

Get daily articles and news delivered to your email inbox

Leave a Comment