What's Michael Jordan's Hang Time on Earth & Beyond?

What’s Michael Jordan’s hang time? The man could defy gravity for 0.98 seconds on Earth. But just imagine if he were on the Moon – he’d fly!

If the 80s or 90s ring any basketball memories, I bet you’ve got a soft spot for MJ. The legendary “Air Jordan” – that nickname still resonates, doesn’t it?

Jordan didn’t just earn his Air Jordan title – he owned it! When he took to the skies, it seemed like he magically suspended himself in mid-air, defying all known laws of physics.

Let’s peel back the layers on Jordan’s uncanny ability to hang time and tease out the physics behind it.

A closer look at human hang time and the equation behind it

“I don’t know about flying, but sometimes it feels like I have these little wings on my feet.”

These iconic words, uttered by Air Jordan himself, captured the essence of his incredible feats. And the mind-boggling part is, for a fleeting moment, he actually did fly. Awestruck fans were left picking up their jaws off the floor, night after night.

Now, as much as we’d love to believe in Jordan’s wings, old Isaac Newton and high school physics remind us that what goes up must, inevitably, come back down. The instant you leap, gravity starts reeling you back to the ground.

Basic physics gives us this nugget of wisdom: $d = -\dfrac{1}{2} \times g \times t^{2}$

This formula tells us the time it takes for something to fall a specific distance ‘d’ above the ground. Here, ‘g’ is Earth’s gravitational acceleration at -32 feet/sec $^{2}$ , and ‘t’ is the time it takes to fall the distance ‘d.’

Important Note: An object’s speed ramps up by 32 feet/sec every second it’s airborne. So, if something’s going at 100 feet/sec initially, after 1 second, it would be zooming at 132 feet/sec. In simple words, due to Earth’s gravity, the object zips from 100 feet/sec to 132 feet/sec in just 1 second.

Now, let’s decipher this hang time enigma! First off, we need to tweak our equation slightly to solve for ‘t’:

$t = \sqrt{-\dfrac{2d}{g}}$

Remember, the time an object spends rocketing upwards equals the time it takes to fall. That’s the crux of hang time.

In essence, a person’s hang time is the total time they’re hovering in the air. To factor this in, we’ll double the height ‘d’ in our equation:

$t = 2 \times \sqrt{-\dfrac{2d}{g}} = \sqrt{-\dfrac{8 \times d}{g}}$

To make things easier, let’s switch from feet to inches, as vertical jumps are measured that way:

$t = \sqrt{\dfrac{8 \times d}{32 \times 12}} = \sqrt{\dfrac{d}{48}}$

Michael Jordan’s hang time calculation on Earth

Students at the University of North Carolina took it upon themselves to measure Jordan’s leaps in various scenarios. Here’s what they found:

Standstill (no basketball): 35.93 inches
Running head start (no basketball): 45.76 inches
During a one-handed dunk: 41.70 inches
During a two-handed dunk: 40.93 inches

Time to throw these jump values into our equation to crunch out his hang time: $t = \sqrt{\dfrac{d}{48}}$

Jump scenario	Vertical jump height	Hang time
Standstill (no ball)	35.93 inches	0.86 seconds
Running head start (no ball)	45.76 inches	0.98 seconds
During a one-handed dunk	41.70 inches	0.93 seconds
During a two-handed dunk	40.93 inches	0.92 seconds

Turns out, MJ’s peak hang time fell just short of 1 second. It might not sound like a lot, but how about we compare it to your average Joe?

A typically spry guy in his 20s can boast an 18-inch vertical, landing him a hang time of 0.61 seconds.

While 0.61 seconds might not seem a far cry from Jordan’s best, bear in mind that in the adrenaline-fuelled world of sports, every tenth of a second is a deal breaker.

Important Note: We all share the same acceleration due to gravity. Some of us simply spring higher than others. How long you float in the air directly ties into the height of your leap. The higher you jump, the longer you stay in the air.

At the end of the day, Jordan doesn’t sidestep the same laws of physics that bind you and me. MJ dazzles us with the illusion of flight thanks to a few tricks up his sleeve:

He cradles the ball for longer before releasing his shot or dunking. It’s only on the way down that he lets go.
He tucks his legs up in mid-air, making it seem like he’s hovering higher than he actually is.
He modifies his center of gravity by coiling and uncoiling his body in the air, ball in hand.

Michael Jordan’s 1988 free throw dunk hang time

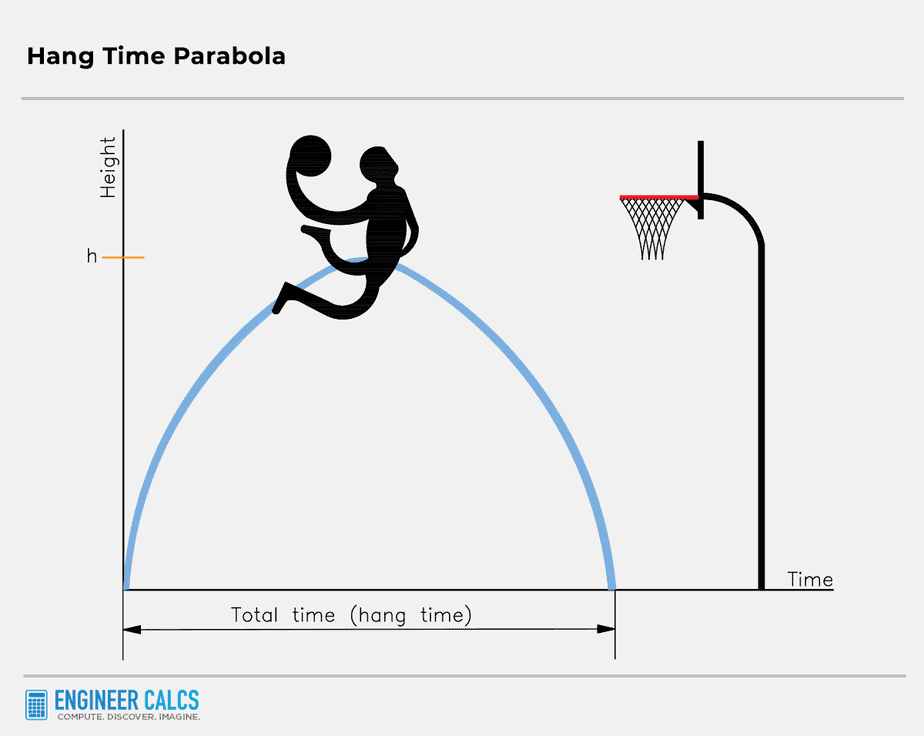
The 1988 free throw dunk is a vivid demonstration of Jordan’s extraordinary hang time. Unfortunately, without solid data on his maximum jump height, we can’t pin down the hang time with precision.

But let’s give it a shot, modeling Jordan’s hang time using this handy equation:

h = ho +vo(t) + 1/2gt $^{2}$ , where,

h: peak height of the falling object
ho: initial height of the object from the surface
vo: object’s initial velocity
t: time the object spends in the air (in seconds)
g: gravitational acceleration

This quadratic equation shows a parabolic relationship between height and air time, with the y-axis standing for jump height and the x-axis for time.

The peak of the parabola corresponds to Jordan’s maximum altitude. A closer peek at the x-intercepts, which signify his take-off and landing points, reveals his hang time – simply the difference between the two intercepts.

In this legendary dunk, Jordan didn’t reach his full jump height. Instead, he channeled his energy to thrust himself forward as well, spanning almost 15 feet toward the hoop from the free-throw line.

Since you can’t maximize both vertical and horizontal jump distances at the same time, Jordan’s mind-blowing hang time lands at a respectable 0.98 seconds.

Important Note: There’s a commonly held belief that the maximum human hang time caps at around 1 second. This limitation is thought to be purely biological.

But hold your horses! If we use our trusty equation $t = \sqrt{\dfrac{d}{48}}$ , we find it’s feasible to have a hang time exceeding 1 second. Picture this: If a jumper has a 50-inch vertical, they would clock a hang time of 1.02 seconds. In the future, who knows? Maybe, thanks to the wonders of bioengineering, someone will pull off a mind-boggling 70+ inch vertical leap.

Michael Jordan’s Hang Time in Outer Space: Let’s Go Wild

Alright, time for some fun! I mean, who wouldn’t want to see how His Airness would fare on different planetary objects? I’m talking about taking Michael Jordan’s hang time to extraterrestrial extremes. After all, even the undisputed king of basketball was bound by Earth’s gravity.

But don’t fret, MJ aficionados! A bit of number crunching allows us to explore just how high Air Jordan could vault on other celestial bodies. Let’s presume Jordan jumps with the same vigor he did on Earth, using his jaw-dropping 45.76-inch vertical as our benchmark.

Let’s see how Jordan would fare on Jupiter, for instance. With a gravity of 24.79 m/sec $^{2}$ , Earth’s 9.81 m/sec $^{2}$ gravity seems puny in comparison. So, Earth’s gravity is just 9.81/24.79 = 0.40 of Jupiter’s gravity.

So, the numbers reveal that Jordan’s vertical jump and hang time on Jupiter would come out as:

45.76 inches x 0.40 = 18.3 inch vertical
0.98 seconds x 0.40 = 0.39 seconds of hang time

Even Air Jordan wouldn’t set the court on fire on Jupiter. But don’t get disheartened about MJ’s celestial capabilities just yet.

Just dream about the wonders on other cosmic bodies! Can you see MJ defying gravity on the Moon or Pluto? With less gravitational resistance, he’d truly be in a league of his own.

For the sheer thrill of it, here are a few more calculated numbers:

Solar system object	Gravity (m/s²)	Jump height (inches)	Hang time (seconds)
Earth	9.81	45.76	0.98
Venus	8.87	50.61	1.26
Mars	3.72	120.67	3.00
Jupiter	24.79	18.11	0.45
Saturn	10.44	43.00	1.07
Neptune	11.15	40.26	1.00
Pluto	0.62	724.04	18.02
Moon	1.62	277.10	6.90
Sun	274	1.64	.04

“What’s Michael Jordan’s hang time?” wrap up

Jordan famously said,

“I mean we all fly. Once you leave the ground, you fly. Some people fly longer than others.”

And boy, was he on point.

Jordan was, without a doubt, an extraordinary athletic powerhouse. Yet, even the iconic MJ couldn’t hover in mid-air for more than a fleeting moment.

But oh, what a spectacle it was! Seeing him glide through the air, reaching heights that seemed impossible, we were witnesses to the stuff of dreams. Time appeared to freeze as he elegantly soared across the court, his trademark tongue playfully sticking out. It was pure, unfiltered poetry in motion.

Now, just imagine Jordan weaving his magic on our moonlit neighbor. That would be a sight for the ages!

What do you think is the most impressive about Jordan’s leaping ability? Do you believe we’ll ever see a human hang in the air for more than 1.5 seconds?

SUBSCRIBE TO ENGINEER CALCS NEWSLETTER

Get daily articles and news delivered to your email inbox

haxor noname

What’s Michael Jordan’s Hang Time on Earth & Beyond?